Exercise 1.4
Exercise 1.4
1) Evaluate:
Solution:
a) |–2| + 4 = 2 + 4 = 6
b) |–5| + |–2| –3 = 5 + 2 – 3 = 4
c) 2 + |–3| – |–5| = 2 + 3 – 5 = 0
d) |3 – |–5|| = |3 – 5| = |–2| = 2
2) Let (i) x=2,y=3
(ii) x=2, y=-3 verify each of the followings:
Solution for (i):
Here, x = 2 and y = 3.
(a) |x|+|y| ≤ |x+y|
|x+y| = |2+3| = 5
And |x| + |y| = |2| + |3| = 5
Hence, |x|+|y| = |x+y|
(b) |x – y| ≥ |x|–|y|
|x – y| = |2 – 3| = 1
And |x|–|y| = |2|–|3| = – 1
Hence, |x – y| > |x|–|y|
(c) |xy| = |x|.|y|
|xy| = |2.3| = |6| = 6
|x|.|y| = |2|.|3| = |6| = 6.
Hence, |xy| = |x|.|y|
(d) $\[\left| {\left.
{\frac{x}{y}} \right|} \right. = \frac{{|x|}}{{|y|}}\]$
Or, $\left| {\frac{{\rm{x}}}{{\rm{y}}}} \right|$ = $\left|
{\frac{2}{3}} \right| = \frac{2}{3}$ and $\frac{{\left| {\rm{x}}
\right|}}{{\left| {\rm{y}} \right|}} = \frac{{\left| 2 \right|}}{{\left| 3
\right|}} = \frac{2}{3}$
Hence, $\left| {\frac{{\rm{x}}}{{\rm{y}}}} \right| =
\frac{{\left| {\rm{x}} \right|}}{{\left| {\rm{y}} \right|}}{\rm{\: }}$
Solution for (ii):
Here, x = 2 and y = – 3
(a) |x|+|y| ≤ |x+y|
|x+y| = |2 – 3| = |–1| = 1
And |x|–|y| = |2| + |–3| = 2 + 3 = 5
Hence, |x+y| < |x|+|y|.
(b) |x – y| ≥ |x|–|y|
|x – y| = |2+3| = 5
And |x|–|y| = |2|–|–3| = 2 – 3 = – 1
Hence, |x – y| > |x|–|y|
(c) |xy| = |x|.|y|
|xy| = |2.(–3)| = |–6| = 6
|x|.|y| = |2|.|–3| = |6| = 6.
Hence, |xy| = |x|.|y|
(d) $\[\left| {\left.
{\frac{x}{y}} \right|} \right. = \frac{{|x|}}{{|y|}}\]$
Or, $\left| {\frac{{\rm{x}}}{{\rm{y}}}} \right|$ = $\left|
{\frac{2}{{ - 3}}} \right| = \frac{2}{3}$ and $\frac{{\left| {\rm{x}}
\right|}}{{\left| {\rm{y}} \right|}} = \frac{{\left| 2 \right|}}{{\left| { - 3}
\right|}} = \frac{2}{3}$
Hence, $\left| {\frac{{\rm{x}}}{{\rm{y}}}} \right| =
\frac{{\left| {\rm{x}} \right|}}{{\left| {\rm{y}} \right|}}{\rm{\: }}$
3) Solve the
following inequalities:
Solution:
a) x– 1 > 2
Solution:
x– 1 > 2
or, x – 1 + 1>2+1
So, x >3
b) x – 3 ≤ 5
Solution:
x – 3 ≤ 5
or, x – 3 + 3 ≤ 5 + 3
or, x ≤ 8
c) – 1 < x –
2 < 3
– 1 < x – 2 < 3
Or, – 1 + 2 < x – 2 + 2< 3 +2
Or, 1 < x < 5
d) – 3 ≤ 2x – 1
≤ 5
Solution:
– 3 ≤ 2x – 1 ≤ 5
or, – 3 + 1 ≤ 2x – 1 + 1 ≤ 5 + 1
or, – 2 ≤ 2x ≤ 6
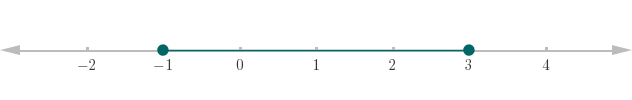
So, – 1 ≤ x ≤ 3.
e. x2 –
2x > 0
Solution:
x2 – 2x > 0
Corresponding equation is x2 – 2x = 0
or, x(x – 2) = 0
So, x = 0, 2.
Let us see the following possible intervals and the sign of
x(x – 2) in three intervals.
Sign of→
|
Intervals |
X |
X – 2 |
X(x – 2) |
|
– ∞ to 0 0 to 2 2 to ∞ |
– + + |
– – + |
+ – + |
From the above table, the possible intervals are (– ∞, 0)
and (2, ∞).
So, the required solution is x ԑ (– ∞, 0) U (2, ∞).
f) 6 + 5x – x2
≥ 0
Solution:
6 + 5x – x2 ≥ 0
(or x2 – 5x – 6 ≤ 0)
Corresponding equation is 6 + 5x – x2 = 0
Or, 6 + 6x – x – x2 = 0
Or, 6(1 + x) – x (1+x) = 0
Or, (1+x)(6 – x) = 0
Or, – (x+1)(x – 6) = 0
So, x = 6, –1.
Let us see the following possible intervals and the sign of
–(x – 6)(x + 1) in these intervals.
Sign
of→
|
Intervals |
(x – 6) |
(x + 1) |
– (x – 6)(x + 1) |
|
– ∞ to – 1 – 1 to 6 6 to ∞ |
– – + |
– + + |
– + – |
From the above table the possible interval is [–1, 6].
So, required solution is x ԑ [–1, 6].
g) $\frac{{{\rm{x}}\left(
{{\rm{x}} + 2} \right)}}{{{\rm{x}} - 1}}$ ≤ 0
Solution:
$\frac{{{\rm{x}}\left( {{\rm{x}} + 2} \right)}}{{{\rm{x}} -
1}}$ ≤ 0
Corresponding equation is $\frac{{{\rm{x}}\left( {{\rm{x}} +
2} \right)}}{{{\rm{x}} - 1}}$ = 0
Or, x (x + 2) = 0
So, x = 0, –2.
Also, the point x = 1.
Let us see the following possible intervals and the sign of
$\frac{{{\rm{x}}\left( {{\rm{x}} + 2} \right)}}{{{\rm{x}} - 1}}$ in three
intervals.
Sign of→
|
Intervals |
x |
X + 2 |
$\frac{1}{{{\rm{x}}
- 1}}$ |
$\frac{{{\rm{x}}\left(
{{\rm{x}} + 2} \right)}}{{{\rm{x}} - 1}}$ |
|
– ∞
to – 2 – 2 to
0 0 to 1 |
– – + |
– + + |
– – – |
– – |
From the table, possible intervals are (–∞, –2] and
[0, 1].
So, the required solution is (–∞, –2] U [0, 1).
4. a) Let A=[-3,1]
and B=[-2,4]. Perform the indicated operations:
Solution:
A = [–3, 1) and B[–2, 4]
Now,
i) A U B
A U B = [–3, 1) U [–2, 4]
= {x: –3 ≤ x < 1} U {x: –2 ≤ x ≤ 4} = (x: –3 ≤ x ≤ 4} =
[–3, 4]
ii) A ∩ B
A ∩ B = [–3, 1) ∩ [–2, 4]
= {x: –3 ≤ x < 1} ∩ {x: –2 ≤ x ≤ 4}
= {x: –2≤x<1}
= [–2, 1)
iii) A – B
A – B = [–3, 1) –[–2, 4]
= {x: –3 ≤ x <1} – {x: –2 ≤ x ≤ 4} = {x: –3 ≤ x < –2}
= [–3, –2)
iv) B – A
B – A = [–2, 4] – [–3, 1]
= {x: –2≤x≤4} – {x: –3≤x<1} = {x:1≤x≤4} = [1, 4]
(b) If A = (–1, 4)
and B[3, 5], find A U B, A ∩ B and A – B.
A = (–1, 4) and B[3, 5]
Now,
A U B = (–1, 4) U
[3, 5)
= {x: –1< x < 4}
U{x: 3≤ x < 5}={x: -1<x<5}=(-1,5)
A ∩ B = (–1, 4) ∩
[3, 5)
= {x: –1< x < 4} ∩ {x: 3≤ x < 5} = {x: 3≤x<4} =
[3, 4)
A – B = (–1, 4) –
[3, 5]
= {x: –1 < x <4} – {x: 3 ≤ x < 5} = {x: –1 < x
< 3} = (–1, 3)
5) Write the
following without using the absolute sign:
(a) |x| < 4
Solution:
|x| < 4
So, – 4 < x < 4.
(b) |x – 3| < 2
Solution:
|x – 3| < 2
= – 2 < x – 3 < 2
= – 2 + 3 < x – 3 + 3 < 2 + 3
So, 1 < x <5.
(c) |2x + 1| ≤ 3
Solution:
|2x + 1| ≤ 3
= – 3 ≤ 2x + 1 ≤ 3
= – 3 – 1 ≤ 2x + 1 – 1 ≤ 3 – 1
= – 4 ≤ 2x ≤ 2
So, – 2 ≤ x ≤ 1
(d) |2x – 1} ≤ 5
Solution:
|2x – 1} ≤ 5
= – 5 ≤ 2x – 1 ≤ 5
= – 5 + 1 ≤ 2x – 1 + 1 ≤ 5 + 1
= – 4 ≤ 2x ≤ 6
So, – 2 ≤ x ≤ 3
6) Rewrite the
following inequalities using absolute sign:
(a) – 5 < x < 7
Solution:
– 5 < x < 7
= – 5 – 1< x – 1 < 7 – 1
= – 6 < x – 1 < 6
So, |x – 1| < 6.
(b) – 3 ≤ x
≤ – 1
Solution:
– 3 ≤ x ≤ – 1
= – 3 + 2 ≤ x + 2 ≤ – 1 + 2
= – 1 ≤ x + 2 ≤ 1
So, |x + 2| ≤ 1
(c) – 3 < x < 4
Solution:
– 3 < x < 4
= – 6 < 2x < 8
= – 6 – 1 < 2x – 1 < 8 – 1
= – 7 < 2x – 1 < 7
So, |2x – 1| < 7
(d) – 4 ≤ x
≤ – 1
Solution:
– 4 ≤ x ≤ – 1
= – 8 ≤ 2x ≤ – 2.
= – 8 + 5 ≤ 2x + 5 ≤ – 2 + 5
= – 3 ≤ 2x + 5 ≤ 3
So, |2x + 5| ≤ 3.
7) Solve the
following inequalities:
(a) |x + 2| < 4
Solution:
|x + 2| < 4
= – 4 < x + 2 < 4
= – 4 – 2 < x + 2 – 2 < 4 – 2
= – 6 < x < 2.
So, the solution is {x: –6 < x < 2} and the graph is:
(b) |x – 1| ≤ 2
Solution:
|x – 1| ≤ 2
= – 2 ≤ x – 1 ≤ 2
= – 2 + 1 ≤ x – 1 + 1≤2 + 1
= – 1 ≤ x ≤ 3.
So, the solution is {x: –1 ≤ x ≤ 3} and the graph is:
(c) |2x + 3| ≤ 1
Solution:
|2x + 3| ≤ 1.
= – 1 ≤ 2x + 3 ≤ 1
= – 1 – 3 ≤ 2x +3 –3 ≤ 1 –3
= – 4 ≤ 2x ≤ –2.
= – 2 ≤ x ≤ – 1
So, the solution is {x: –2 ≤ x ≤ –1} and the graph is:
(d)|x-1|>1
Solution:
Here, two cases arises,
Case I:
When (x – 1) > 0 then.
= x – 1 + 1 > 1 + 1
So, x > 2, i.e. x ԑ (2, ∞)
Case II:
When (x – 1) < 0 then,
= x – 1 < – 1
= x – 1 + 1 < – 1 + 1
So, x < 0 i.e. x ԑ (–∞, 0)
Hence, the required solution of |x – 1| > 1 is {x:x< 0
or x > 2} i.e. x ԑ (–∞, 0) U (2, ∞)
The graph is:
(e) |2x + 1| ≥ 3
Solution:
|2x + 1| ≥ 3
Here, two cases arises
Case I:
When (2x + 1) > 0 then.
(2x + 1) ≥ 3
= 2x + 1 – 1 ≥ 3 – 1
= 2x ≥ 2
So, x ≥ 1 i.e. x ԑ [1, ∞)
Case II:
When (2x + 1) < 0 then,
– (2x + 1) > 3
= 2x + 1 ≤ – 3
= 2x + 1 – 1 ≤ – 3 – 1
= 2x ≤ – 4
So, x ≤ – 2.i.e. x ԑ (–∞, – 2]
Thus, the required solution is {x:x ≤ – 2 or x ≥ 1}
I.e. x ԑ (–∞. – 2] U [1, ∞) and the graph is:
8) Using the properties of real numbers, Prove that:
a)a+b=b+c⇒a=c
Here,
a + b = b + c
= a + b – b = b – b + c
So, a = c
b) ac = bc⇒a=b
Here, ac = bc
= (ac)c – 1 = (bc)c – 1
= a(cc – 1) = b(cc – 1)
[Associativity]
= a.1 = b.1
So, a = b
c)a<b⇒a+c<b+c
Here, a < b.
So, a + c < b + c [adding c on both sides]
d)a<b and c<d⇒a+x<b+d
Here, a < b.
= a – b < 0 ….(i)
And c < d
= c – d < 0 ….(ii)
Adding (i) and (ii) we have,
a – b + c – d < 0
= a – b + b + c – d + d < b +
d [Adding b + d on both sides]
So, a + c < b + d
(e) a>b and c<0⇒ac<bc
Here, a > b
= (a –b)c <
0.c
[c<0]
Or, ac – bc< 0
Or, ac < bc
(f)a>b and c>0 ⇒ $\frac{{\rm{a}}}{{\rm{c}}}$>$\frac{{\rm{b}}}{{\rm{c}}}$
Here, a > b
= a – b > 0
= $\frac{{{\rm{a}} -
{\rm{b}}}}{{\rm{c}}}$>$\frac{0}{{\rm{c}}}$ [c >0]
= $\frac{{\rm{a}}}{{\rm{c}}}$ –
$\frac{{\rm{b}}}{{\rm{c}}}$> 0
= $\frac{{\rm{a}}}{{\rm{c}}}$ – $\frac{{\rm{b}}}{{\rm{c}}}$
+ $\frac{{\rm{b}}}{{\rm{c}}}$>$\frac{{\rm{b}}}{{\rm{c}}}{\rm{\:
}}$
$\frac{{\rm{b}}}{{\rm{c}}}$ [Adding
on both sides]
So, $\frac{{\rm{a}}}{{\rm{c}}}$>$\frac{{\rm{b}}}{{\rm{c}}}$